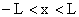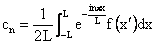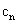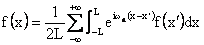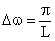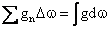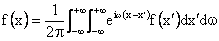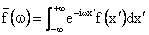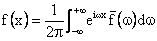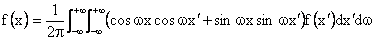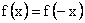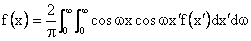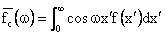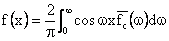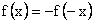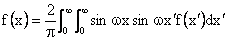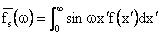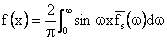



















|

|
| Engineering Mathematics | |
| Special Functions | Differential Equations | Fourier Series | Laplace Transforms | Probability and Random Processes | Linear Systems | Useful Integrals | Vector Identities | |

 |
|
Fourier Series Summary of Fourier Transform Properties Consider the Fourier Exponential Series in the region
Replacing the expression for the coefficient
In the last step we have defined
As
Define Fourier Transform (Exponential)
The inverse transform is
The above two equations are a Fourier Exponential Transform Pair. Fourier Integral Representation (FIR) may be restated as
or
For even functions (i.e.
Definition: Fourier-Cos Transform Pair:
For odd functions (i.e.
Definition: Fourier-Sin Transform Pair:
|

 |