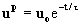


















|
|
Aerosol Kinetics Terminal Velocity For a particle starting from rest, the solution to equiaton 2 is given as
where uƒ is assumed to be a constant vector. For uƒ=0 and large t, the terminal velocity of particle ut is given by
Table 7: Relaxtion time τ for a unit density particle in the air(p=1 atm, T=20°C)
Stopping Distance In the absence of gravity and fluid flow, for a particle with an initial veolocity of u
where xp is the position of the particle. As t→&infin, up→0 and
is known as the stopping distance of the particle. For an initial veolocity of 1000 cm/s, the stop distance for various particles is listed in Table 7. |


|


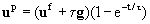
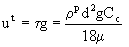
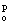 ,
the solution to 2 is given by
,
the solution to 2 is given by