| Electrodynamics
Coulombís Law
Combining (5) and (12), we find
 ,†††††††† (cgs)††††††††††††††††††††††††††††††††††††††††††††††††††††††††††††† †††† (13) ,†††††††† (cgs)††††††††††††††††††††††††††††††††††††††††††††††††††††††††††††† †††† (13)
which is Coulombís law for forces between two charge particles.† Note that in the MKS units, 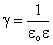 , and the permittivity (dielectic constant) of free space, , and the permittivity (dielectic constant) of free space, 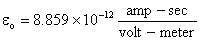 . .
 ,††††† (MKS). †††††††††††††††††††††††††††††††††††††††††††††††††††††††† †††††(14) ,††††† (MKS). †††††††††††††††††††††††††††††††††††††††††††††††††††††††† †††††(14)
Field Charging
When a particle is in an electric field, the particle acquires charges due to collisions with ions, which are moving along the lines of force that intersect the particle surface. This process is known as field charging. The number of charges accumulated by the particle is given by
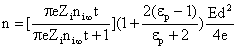 ,† (cgs), †††††††††††††††††††††††††††††††††††† †††††(15) ,† (cgs), †††††††††††††††††††††††††††††††††††† †††††(15)
where 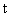 †is time, †is time,  †is the mobility of ions, †is the mobility of ions,  †is the ions concentration far from the particle, †is the ions concentration far from the particle,  †is the electronic charge, †is the electronic charge, 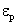 †is the dielectric constant of the particle, and †is the dielectric constant of the particle, and 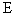 †is the electric field intensity. †is the electric field intensity.
For sufficiently large time,
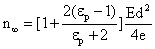 †††††††† as †††††††† as  .†††††††††††††††††††††††††††††††† ††† †††††† †††††(16) .†††††††††††††††††††††††††††††††† ††† †††††† †††††(16)
The factor 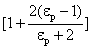 †is a measure of distortion of the electrostatic field by the particle.† The factor varies between 1 and 3 for †is a measure of distortion of the electrostatic field by the particle.† The factor varies between 1 and 3 for 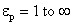 .† For dielectric materials, the values of .† For dielectric materials, the values of 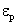 †usually are less than 10.† †usually are less than 10.† 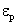 †is 4.3 for quartz and 2.3 for benzene. †is 4.3 for quartz and 2.3 for benzene.
Diffusion Charging
Even in the absence of an external electric field, particles exposed to an ion cloud become charged. Ions will collide with the particle due to their thermal motions. As the particle becomes charged, it will repel ions of the same sign and leads to a non-homogenous distribution of ions in its neighborhood. After time 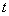 , the number of charge acquired by the particle is given by , the number of charge acquired by the particle is given by
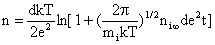 ,†††††††††††††††† (cgs)†††††† †††† ††††††††††† †††††(17) ,†††††††††††††††† (cgs)†††††† †††† ††††††††††† †††††(17)
where 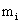 †is the ionic mass and †is the ionic mass and  †is the mean velocity with which the ions strike the particle surface. †is the mean velocity with which the ions strike the particle surface.
As 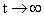 , the number of charge (according to the formula) also approaches infinity, which is not correct. However, for typical values of , the number of charge (according to the formula) also approaches infinity, which is not correct. However, for typical values of  , the equation gives reasonable results, especially for particles, which are smaller than the mean free path. , the equation gives reasonable results, especially for particles, which are smaller than the mean free path.
|


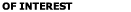






















 ,†††††††† (cgs)††††††††††††††††††††††††††††††††††††††††††††††††††††††††††††† †††† (13)
,†††††††† (cgs)††††††††††††††††††††††††††††††††††††††††††††††††††††††††††††† †††† (13)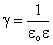 , and the permittivity (dielectic constant) of free space,
, and the permittivity (dielectic constant) of free space, 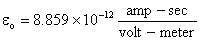 .
. ,††††† (MKS). †††††††††††††††††††††††††††††††††††††††††††††††††††††††† †††††(14)
,††††† (MKS). †††††††††††††††††††††††††††††††††††††††††††††††††††††††† †††††(14)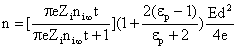 ,† (cgs), †††††††††††††††††††††††††††††††††††† †††††(15)
,† (cgs), †††††††††††††††††††††††††††††††††††† †††††(15)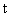 †is time,
†is time,  †is the mobility of ions,
†is the mobility of ions,  †is the ions concentration far from the particle,
†is the ions concentration far from the particle,  †is the electronic charge,
†is the electronic charge, 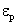 †is the dielectric constant of the particle, and
†is the dielectric constant of the particle, and 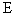 †is the electric field intensity.
†is the electric field intensity.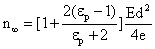 †††††††† as
†††††††† as  .†††††††††††††††††††††††††††††††† ††† †††††† †††††(16)
.†††††††††††††††††††††††††††††††† ††† †††††† †††††(16)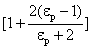 †is a measure of distortion of the electrostatic field by the particle.† The factor varies between 1 and 3 for
†is a measure of distortion of the electrostatic field by the particle.† The factor varies between 1 and 3 for 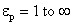 .† For dielectric materials, the values of
.† For dielectric materials, the values of 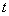 , the number of charge acquired by the particle is given by
, the number of charge acquired by the particle is given by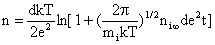 ,†††††††††††††††† (cgs)†††††† †††† ††††††††††† †††††(17)
,†††††††††††††††† (cgs)†††††† †††† ††††††††††† †††††(17)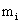 †is the ionic mass and
†is the ionic mass and  †is the mean velocity with which the ions strike the particle surface.
†is the mean velocity with which the ions strike the particle surface.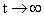 , the number of charge (according to the formula) also approaches infinity, which is not correct. However, for typical values of
, the number of charge (according to the formula) also approaches infinity, which is not correct. However, for typical values of  , the equation gives reasonable results, especially for particles, which are smaller than the mean free path.
, the equation gives reasonable results, especially for particles, which are smaller than the mean free path.